UPSC Geo-Scientist and Geologist Exam Papers 2018 : Geo-Physics Paper - I

(Download) UPSC: Geologist Examination Papers-2018

Exam Name : UPSC Geo-Scientist and Geologist Exam 2018

Subject : Geophysics Paper - I

Year : 2018

GEOPHYSICS
Paper I

Time Allowed : Three Hours

Maximum Marks : 200

QUESTION PAPER SPECIFIC INSTRUCTIONS

Please read each of the following instructions carefully before attempting questions.

There are TEN questions divided under TWO Sections.

Candidate has to attempt SIX questions in all.

Questions No. 1 and 6 are compulsory.

Out of the remaining EIGHT questions, FOUR questions are to be attempted choosing TWO from each Section.

The number of marks carried by a question / part is indicated against it.

Attempts of questions shall be counted in sequential order. Unless struck off, attempt of a question shall be counted even if attempted partly.

Any page or portion of the page left blank in the answer-book must be clearly struck off. Answers must be written in ENGLISH only.

Neat sketches may be drawn to illustrate answers, wherever required.

Unless otherwise mentioned, symbols and notations have their usual standard meanings. Assume suitable data, if necessary and indicate the same clearly.

SECTION ‘A’

1. (a) Draw and explain different body-wave phases from an earthquake passing through the Earth.
1. (b) An electromagnetic sounding was carried in three decades i.e., 10 kHz - 10 Hz. Observations indicated that

(i) 10 kHz - 1000 Hz corresponds to 3D features
(ii) 1000 Hz - 100 Hz corresponds to 2D features
(iii) 100 Hz - 10 Hz corresponds to ID features
Draw the ellipse of polarization for representative frequencies in these three decades.

1. (c) If continental crust of 29 km thickness and oceanic crust are in isostatic equilibrium at mean sea level what will be thickness of the oceanic crust of density 2.95 gm/cm3, below 05 km ocean water, if density of the continental crust is 2.83 gm/cm3, density of mantle is 3.33 gm/cm3 and density of water is 1.0 gm/cm3 ?
1. (d) Inversion of the following 3-layered earth was performed :

Layer	Resistivity (ohm - m)	Thickness (m)
1	400	30
2	40	10
3	1000	-

The obtained correlation matrix is as:

Resistivity of layer 1	Resistivity of layer 2	Resistivity of layer 3	Thickness of layer 1	Thickness of layer 2
+ 1.0	+ 0.21	+ .011	+ 0.05	+ 0.03
	+ 1.0	+ 0.24	+ 0.20	+ 0.98
		+ 1.0	+ 0.16	- 0.2
			+ 1.0	+ 0.2
				+ 1.0

Each layer is assumed to be electrically homogenous and isotropic.
Comment on the resolution of the layer parameters, eigenvalues an Also draw the histogram of the obtained layer parameters after inversion.

2. (a) State three main sources of Earth's surface heat flow. Define decay depth of penetration and delay time for thermal wave penetrating the subsurface. Calculate the ratio of decay depth for annual temperature variation to decay depth for daily variations.
2. (b) Calculate the present day relative motion at 28° S, 71° W on the Peru-Chile Trench using the Nazca-South America rotation pole (56-0° N, 94.0° W) having angular velocity 7.2x10^-7 deg/yr. Assume the radius of the Earth to be 6371 km.
2. (c) In a subduction zone seismometers are planted in a direction perpendicular to the trench. For an earthquake arrival times are plotted for the receivers in the dip-direction. The estimated velocity from travel time plot for the top layer and subducting layer is 3 km/s and 6 km/s, respectively. What will be the dip angle of the subducting plate if the critical angle is 20° ? (Assume each layer is homogeneous and isotropic)

3. (a) Assuming two-layered horizontally stratified homogeneous Earth as crust and mantle, draw the ray-path and derive expression of travel-time for refracted seismic waves. How you can estimate the velocity of the layers ? Explain different steps to estimate the thickness of the first layer, i.e. crust.
3. (b) What is emperical relation between maximum intensity with surface wave magnitude for shallow focus earthquake. Derive expression for the maximum magnitude of the earthquake using Gutenberg-Richter relationship. Calculate number of earthquakes of zero magnitude assuming constant a=8.
3. (c) In a spherically symmetric planet of radius 6400 km velocity varies as a function of only radius. A seismic wave emerges the surface of the planet at an angle 30o from the vertical. The seismic wave velocity is 6 km/s. The deepest point of the ray touches the top of the core of the planet (having seismic velocity 9 km/s). What will be the depth of the core of the planet from the surface of the planet ?

4. (a) Derive the expression for a gravity anomaly due to a buried sphere with its centre at depth z below the surface. The ratio of gravity anomalies for distances 4 km and 10 km along a profile is 8. Assuming a spherical target, calculate the depth of the causative source.
4. (b) State the boundary condition in terms of electrical potential and current density to be satisfied at the interface separating two electrically homogenous and isotropic medium. Explain why these need to be satisfied. A current source in medium (2) of resistivity 1000 Ohm-m comes in contact with the interface at an angle of 45° and enters medium (1) of resistivity 100 Ohm-m. Calculate

(i) reflection coefficient,
(ii) the angle at which it enters medium (1), and
(iii) comment whether the current line would bend towards the normal or away from the normal.
4. (c) Magnetic measurements have been made on a basalt flow at present at 17° N 20° E. The angle of inclination of the remanent magnetization of this basalt is 45°. How much it has moved from the magnetic latitude at the time of magnetizati basalt ?

5. (a) Discuss the implication of Ñ.D = P_s(where symbols have their original meaning), when time varying natural source electromagnetic measurements are made in perpendicular direction over a swamp embedded in a highly resistive host rock.
5. (b) With the help of neat diagram discuss the variation of magnetic power with frequency (in the range of 10 kHz - 10000 seconds). Assume that the magnetic field has external origin.
5. (c) Find the model estimate for first five iterations of the following equation 2m³= 16. Assume initial guess of 1.0.

SECTION 'B'

6. (a) Write Maxwell's equations in free space and obtain wave equation for electric field vector. Hence, show that light is an e.m. wave.
6. (b) What is ionization potential ? The ionization potentials of most of the gases in Earth's atmosphere are around 15 eV. Will green light (l = 550 nm) ionize these gases ? Take (1 J = 6-24x10¹⁸ eV)
6. (c) Verify Cayley-Hamilton theorem for the matrix

A = [1 2 0, 2 -1 0, 0 0 1]

Also calculate its eigenvalues and corresponding to any one of these values, calculate the eigenvector.
6. (d) What is Brownian motion ? State its three observed characteristics.
(ii) Sedimentation is a familiar example of Brownian motion. Obtain an expression for variation of number density with height under the influence of gravity and diffusion.

7. (a) Determine the roots of the indicial equation around the origin for the differential equation

7. (b) In a resonant cavity, an e.m. oscillation of frequency 0, dies out as

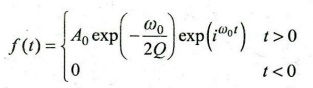
The parameter is a measure of the ratio of the stored energy to energy loss per cycle. Determine the frequency distribution of the oscillation g*(w) g(w), where g(0) is the Fourier transform of f(t). Interpret your result physically
7.(c) The generating function for Bessel function of the first kind is

Obtain the recurrence relation for Jn(x).

8. (a) Write down the expression for Planck's distribution law for black body radiation in terms of frequency and wavelength. Obtain Wien's displacement law.
8. (b) It is observed that vapour pressure curve for a gas on the p-T diagram near the triple point can be fitted with the equation in p= 24.78 -3073 K/T where p is measured
in pascal. Similarly, the solid-vapour equilibrium curve for this gas can be fitted with equation In p = 28.32 - 3764 K / T. Calculate the triple point temperature and pressure.
8. (c) Plot specific Gibbs energy and its first order derivatives as a function of temperature for first and second order phase transitions.

9. (a) A particle having charge q is moving with non-relativistic speed V in Electrostatic and Magnetostatic fields. Show that its

(i) Kinetic energy is constant when it moves in uniform magnetostatic field only. Also, depict its motion.
(ii) Total energy (sum of KE & PE) is conserved in presence of static electric and magnetic fields.
9. (b) Show that the solution of Laplace equation in rectangular coordinate system are harmonic functions.
9. (c) A transmitting antenna is kept on the top of a tower at height 36 m and the receiving antenna is kept at the height 49 m. Calculate the maximum distance between them for satisfactory communication in the line of sight mode. Take radius of the Earth as 6400 km.

10. (a) Obtain the expression for electromagnetic fields at point P located at distance r in space due to a small dipole antenna oriented in z-direction and having the current element Idl. Given that the z-component of the vector potential at point P is

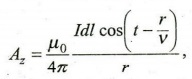
where the symbols have their usual meaning. Also find at what distance the induction field and radiation field become equal.
10. (b) What is the significance of ionosphere for a planet ? Discuss chemical and photochemical processes taking place in the Earth's ionosphere.
(ii) Draw the variation of electron density with altitude for the Earth's ionosphere.
10. (c) What do you understand by the term GPS ? How does it work?
(ii) Can GPS signal be jammed ? Explain.