(FREE) UPSC Current Affairs 2025 PDF
NEW! The Gist (MAY-2025) | E-BOOKS
(IGP) IAS Pre Paper - 2: GS - Basic Numeracy - Average
Basic Numeracy
Average
Average
The average of a given number of quantities of the same kind is expressed as
Average = Sum of the quantities/Number of the quantities
Average is also called the Arithmetic Mean.
Also, Sum of the quantities = Average × Number of the quantities
Number of quantities = Sumof the quantities/Average
If all the given quantities have the same value, then the number itself is the average.
-
If all the given quantities are not all the same, then the average of the given quantities is always greater, then the smallest number and always less than the largest number. Equivalently, atleast one of the numbers is less than the average and atleast one is greater then the average.
- If each of the given quantities is increased by a constant p, then their average is also increased by p.
- If each of the given quantities is decreased by a constant p, then their average is also decreased by p.
- If each of the given quantities is multiplied by a constant p, then their average is also multiplied by p.
- Whenever the given quantities form an arithmetic sequence and if the given quantities has odd terms, then the average is the middle term in the sequence and if the given quantities has even terms, then the average of the sequence is the average of the middle two terms.
-
In order to calculate the weighted average of a set of numbers, multiply each number in the set by the number of times it appears, add all the products and divide by the total number of numbers in the set.
- If the speed of an object from A to B is x km/h and from B to A is y km/h,then the average speed during the whole journey is 2xy/x + y km/h.
- If the average of N1 quantities is x and N2 quantities is y then the average of total (N1 + N2) quantities is given by (N1x+N2y)/N1+N2
Example 1: What is the average of first five even numbers.
Solution. The first prime even numbers are 2, 4, 6, 8, 10
Average =2+4+6+8+10/5 =30/5=6
Example 2: The average of five consecutive even numbers is 50. What is
the largest of these numbers?
Solution. Let the numbers be x – 4, x – 2, x, x + 2, x + 4.
Average = Sum of the quantities/Number of the quantities
=x-4+ x-2+ x+x+2+ x+4/5=50
5x/5=50
x=50
So, the numbers are 46, 48, 50, 52, 54.
The largest of these numbers is 54.
Example 3: Average weight of 32 students of a class is 30.5 kg. If weight of a teacher is also included then average weight is increased by 500 g. What is the weight of the teacher?
Solution. Total weight of 32 students = 30.5 × 32 = 976 kg
Average weigth of (32 students + 1 teacher) = (30.5 + 0.5) = 31 kg
Total weight of (32 students + 1 teacher) = 31 × 33 = 1023 kg
Weight of teacher = (1023 – 976) kg = 47 kg
Example 4: The average salary per head of all the employees of an institution is Rs 60. The average salary per head of 12 officers is Rs 400 and average salary per head of the rest is Rs. 56. Find the total number of employees in the institution.
Solution. Let the total number of employees be x.
Then, 60 = Total salary of all employees/x
60 = 12X400 (x-12)X56/x
60x = 12 × 400 + (x – 12) × 56 = 4800 + 56x – 672
60x – 56x = 4800 – 672
4x = 4128 >> x = 1032
Hence, the total number of employees is 1032.
Example 5: If the average of p and q is 58 and the average of q and 5 is 64, what is the value of s – p?
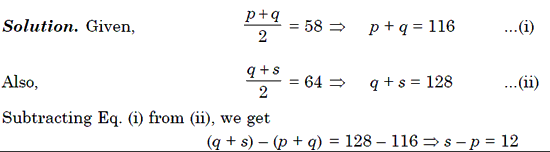
Example 6: 12 men went to a restaurant. 11 of them spent Rs. 5 each
and the 12th person spent Rs. 11 more than the average expenditure of all. Find
the total money spent by them?
Solution. Let the average money spent by the 12 men = Rs. x
Money spent by the 12th man = Rs. (x + 11)
Money spent by the other 11 men = Rs. (11 × 5) = Rs. 55
Total money spent by the 12 men = Rs. (55 + x + 11) = Rs. (x + 66)
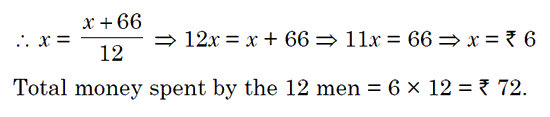
© UPSCPORTAL.COM

