(IGP) IAS Pre Paper - 2: GS - Basic Numeracy - Concepts of Geometry (MCQ -18)

1. Find themeasure of an anglewhich is complement of itself.
(a) 45°
(b) 36°
(c) 42°
(d) 35°

2. An angle is equal to five times its supplement. Find itsmeasure.
(a) 110°
(b) 140°
(c) 150°
(d) 100°

3. The supplement of an angle is one-fourth of itself. Determine the angle and its supplement.
(a) 132°, 48°
(b) 156°, 24°
(c) 118°, 62°
(d) 144°, 36°

4. Two complementary angles differ by 18°, Find the angles.
(a) 42°, 60°
(b) 36°, 54°
(c) 24°, 66°
(d) 18°, 7 2°

5. If two supplementary angles are in the ratio 1 : 5. Find the difference of the angles.
(a) 64°
(b) 90°
(c) 36°
(d) 70°

6. In the given figure, find ÐADB

(a) 132°
(b) 144°
(c) 48°
(d) 96°

7. In the following figure, find x

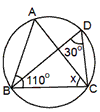
(a) 40°
(b) 25°
(c) 30°
(d) 45°

8. In the following figure, it is given that 0 is the centre of the circle andÐAOC = 140° . Find Ð ABC

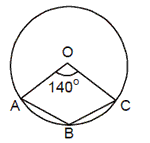
(a) 110°
(b) 120°
(c) 115°
(d) 130°

9. Find x in the given figure

(a) 13 cm
(b) 12 cm
(c) 16 cm
(d) 15 cm

10. In the figure below, PQ = QS, QR = RS and SRQ = 100° . FindÐQPS

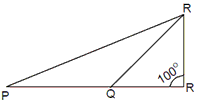
(a) 20°
(b) 40°
(c) 15°
(d) 735°

11. Find x in the adjoining figure

(a) 1.6 cm
(b) 2.2 cm
(c) 3 cm
(d) 2.6 cm

12. In the given figure, AB, CD, EF are concurrent straight lines which pass through O. If Ð AOE = 90° and x : y = 21, find Ð DOB and Ð COF.
(a) 50°, 100°
(b) 30°, 60°
(c) 45°, 90°
(d) 25°, 50°

13. In the given figure, AB || CD and EF || GH. Find the relation between a and b.

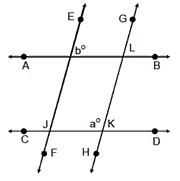
(a) 2a + b=180°
(b) a + b = 180°
(c) a – b = 180°
(d) a + 2b = 180°

14. In the given figure, if l || m, find the value of x in degree.

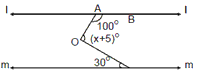
(a) 105°
(b) 100°
(c) 110°
(d) 115°

15. In the given figure, AB || CD and they cut PQ and QR at E, F and G, H respectively. Ð PEB = 70° and Ð QHD =140° and Ð PQR = x. Find the value of x in degree.

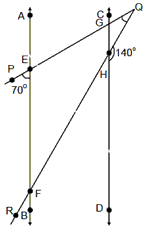
(a) 20°
(b) 30°
(c) 24°
(d) 32°

16. In the given figure, AB ^CD; AP || CD, Ð CBP = 142° Find Ð ABP and ÐAPB.

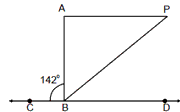
(a) 52°, 38°
(b) 56°, 34°
(c) 51°, 39°
(d) 57°, 33°

17. In the figure, Ð CAB = 72°, ÐCBA = 74° and Ð CED = 112° Find Ð CDE.

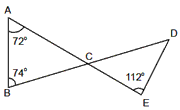
(a) 34°
(b) 33°
(c) 35°
(d) 38°

18. In the given figure, CD || AB. Find y.

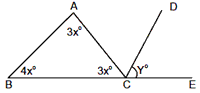
(a) 79°
(b) 72°
(c) 74°
(d) 77°

19. In D ABC, DE || BC, AD = 2.5 cm, DB = 5 cm, AE = 2 cm and BC = 9 cm. Find EC and DE. A 2.5 cm 2 cm

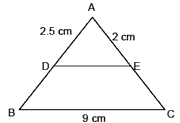
(a) 4 cm, 3 cm
(b) 5 cm, 3 cm
(c) 2 cm, 4 cm
(d) 4 cm, 5 cm

20. In the figure, AB || DE and AC = 2 cm, CE = 6 cm and DB = 12 cm. Calculate DC.

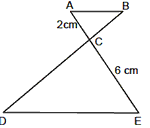
(a) 6 cm
(b) 9 cm
(c) 12 cm
(d) 3 cm

© UPSCPORTAL.COM